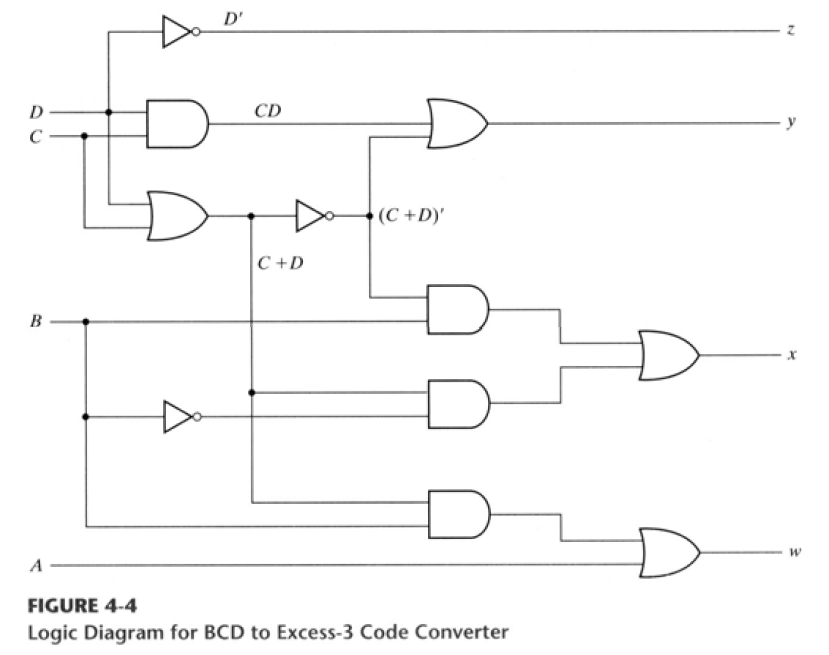
BCD to Excess-3 Conversion Circuit
BCD에서 3-초과 부호로 변환하는 회로
- BCD 코드에 0011을 더한 코드 체계이다.
* BCD Code (URL)
[Digital Logic] BCD Code Representation | BCD 코드 방식
BCD Code Representation BCD 코드 방식 - Binary Coded Decimal의 약자로, 우리말로는 2진화 10진 코드라고 한다. (8421코드라고 하기도 한다.) - 10진수를 이진코드로 표기하는 방법 중 하나로, 장점은 10진수와의
dad-rock.tistory.com
* Excess-3 Code (URL)
Excess-3 - Wikipedia
From Wikipedia, the free encyclopedia Variation to BCD-code where three (11) is added to a binary representation Excess-3, 3-excess[1][2][3] or 10-excess-3 binary code (often abbreviated as XS-3,[4] 3XS[1] or X3[5][6]), shifted binary[7] or Stibitz code[1]
en.wikipedia.org
Specification
Inputs
- 4 Variables (\(A, B, C, D\))
Outputs
- 4 Variables (\(w, x, y, z\))
Truth Table
| Inputs (BCD Code: 0 ~ 9) |
Outputs (Excess-3 Code: 0~9) |
||||||
| \(A\) | \(B\) | \(C\) | \(D\) | \(w\) | \(x\) | \(y\) | \(z\) |
| 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 |
| 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 |
| 0 | 0 | 1 | 1 | 0 | 1 | 1 | 0 |
| 0 | 1 | 0 | 0 | 0 | 1 | 1 | 1 |
| 0 | 1 | 0 | 1 | 1 | 0 | 0 | 0 |
| 0 | 1 | 1 | 0 | 1 | 0 | 0 | 1 |
| 0 | 1 | 1 | 1 | 1 | 0 | 1 | 0 |
| 1 | 0 | 0 | 0 | 1 | 0 | 1 | 1 |
| 1 | 0 | 0 | 1 | 1 | 1 | 0 | 0 |
| Don't Use Below | |||||||
| 1 | 0 | 1 | 0 | X | X | X | X |
| 1 | 0 | 1 | 1 | X | X | X | X |
| 1 | 1 | 0 | 0 | X | X | X | X |
| 1 | 1 | 0 | 1 | X | X | X | X |
| 1 | 1 | 1 | 0 | X | X | X | X |
| 1 | 1 | 1 | 1 | X | X | X | X |
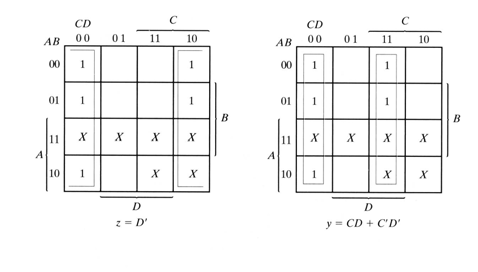
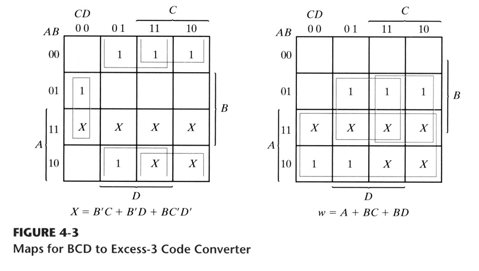
Karnuagh Map Representation & Minimized Boolean Function
Total Cost = 7 ANDs + 3 ORs
※ Cost를 고려한 Minimized Form
\(z = D '\)
\(y = CD + C 'D ' = CD + (C + D) '\)
\(x = B ' \cdot (C + D) + B \cdot (C + D) '\)
\(w = A + BC + BD = A +B \cdot (C + D)\)
\(\therefore\) Total Cost = 4 ANDs + 4 ORs
Circuit Implementation